Solved Problems of Rectilinear Kinematics Continuous Motion
Although this website is dedicated to explaining mathematics, many of you ask me to help you solve physics exercises. The most repeated exercise that you have asked me to do is the uniform rectilinear movement, so I will dedicate this post to explain in detail, step by step, how to solve this type of exercise.
I'm not going to go into terms of physics, such as the difference between position, space traveled, distance, direction, sense … since you can consult them in your book. I will focus on your understanding the procedure to learn how to solve the exercises.
What is Uniform Rectilinear Motion
To understand this type of exercise you have to be clear about what uniform rectilinear motion is.
As its name suggests, we speak of uniform straight-line motion when a body, be it a vehicle, an object, an animal, whatever it is, moves in a straight line at a constant speed:
- Movement: An object moves
- Rectilinear: Straight line
- Uniform: Constant speed
It is important to keep this in mind, because if any of these premises are not met, the formulas of the uniform rectilinear motion would no longer be valid.
Formulas of the Uniform Rectilinear Motion
These three variables intervene in the uniform rectilinear motion:
- Position: Position from which it begins to move (initial position) and the position where it arrives after a while (final position).
- Speed: The speed will always be constant. If it influences his direction
- Time: Instant in which the object begins to move (initial time) or the time it takes to travel a certain distance (final time).
These three variables are related by this formula:
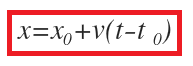
Where:
- X = Final position (meters in the international system)
- Xo = Initial position (meters, in the international system)
- v = Speed (meters/second, in the international system)
- to = Initial time (seconds, in the international system
- t = End time (seconds, in the international system)
Changing Units
We must always work in the same units. We are not obliged to work with the units of the international system, but we do so in order to establish a criteria.
If the statement gives you the time in hours and the distance in kilometers, you have to take into account that the final position will be in kilometers and the speed in km/h.
You also have to be very careful when mixing units in the statement, for example, give you the data in hours but the result you ask in seconds.
You must master perfectly the change of units.
What you can't do, under any circumstances, is mix time and distance units. Never!
Example of Resolved Uniform Rectilinear Motion Exercises
We are going to solve step by step one of the examples of the most typical exercises of uniform rectilinear motion:
Two trains depart from two cities, 500 km apart in a straight line. Train A has a speed of 180 km/h. Train B leaves 1 hour later, in the opposite direction, with a speed of 200 km/h.
- a) How long will it take to get there?
- b) Where will they meet?
- c) How much distance does each one travel?
First of all, you must make an outline of the data that the statement gives you and have very clear what they are asking you. Forget about formulas for the moment:

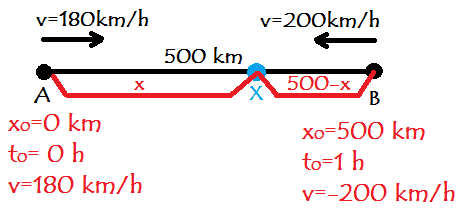
We draw two points A and B separated 500 km, are their corresponding speeds, each with its sense and place more or less where we think they will be, the point x.
Now it is necessary to establish the initial conditions for each train, that is, its initial position, its initial time and the direction of the speed, from our reference system, and according to the signs of the coordinate axes:
Reference of space:
Initially, point A is at point 0 and point B on its right will be at point 500.
If we took point B as 0, point A would be -500,
Time reference:
Train B leaves one hour later, then we start counting when train A leaves. That is to say, the initial time of train A will be 0 and therefore for train B, the time will have already advanced 1 hour when it leaves:
Tren A –> to = 0 h
Tren B –> to = 1 h
Always set to = 0 for the train departing earlier, as this is our reference to when time begins to count. If the statement says that train B leaves 2 hours earlier, then:
Tren A –> to = 2 h
Tren B –> to = 0 h
Direction of speed:
Train A goes to the right, then its sign is positive, according to the coordinate axes.
On the other hand, train B, when going to the left, the speed sign is negative.
We indicate all the data in our scheme:
Now we only have to apply the formula for each train. The only data we don't know are the final position, x, and the final time t:
For train A:
![]()
![]()
For train B:
![]()
![]()
![]()
As it is a meeting point, x is the same in the two equations, so we equal them to clear t, which will also be the same:
![]()
And we clear t:
![]()
With this value of t, we substitute it in either of the two equations. It's easier in the first one:
![]()
Note that I have not worked with the units of the international system, since the statement gave me the data in hours, km and km/h and I have not had to make any change of unit.
Now we can answer all the questions:
a) It will take them 1.84 hours to find each other
b) You will be at point 331,2 km
c) If you notice in the diagram, train A travels x km and therefore, train B travels 500-x. Remember that x is a position, that is, a point. The space traveled is equal to the final position, minus the initial position:
Travelled space= End position – Starting position
To go from point A to point X, train A covers 331.2 km:
Traveled space=331,2-0=331,2 km
To go from point B to point X, train A covers 168.8 km:
Traveled space=500-331,2=168,8 km
Source: https://ekuatio.com/uniform-rectilinear-motion-solving-problems-step-by-step/
0 Response to "Solved Problems of Rectilinear Kinematics Continuous Motion"
Post a Comment